Converse, Inverse, and Contrapositive

Hi, and welcome to this video on mathematical statements! Today, we’ll be exploring the logic that appears in the language of math. Specifically, we will learn how to interpret a math statement to create what are known as converse, inverse, and contrapositive statements. These, along with some reasoning skills, allow us to make sense of problems presented in math. Let’s get started!

Let’s first take a look at a basic statement, which can be either true or false, but never both. For example, a declarative statement pronounces a fact, like “the Sun is hot.” We know this is a statement because the Sun cannot be both hot and not hot at the same time. This declarative statement could also be referred to as a proposition.

Two independent statements can be related to each other in a logic structure called a conditional statement. The first statement is presented with “if,” and is referred to as the hypothesis. The second statement is linked with “then”, and is known as the conclusion. The notation associated with conditional statements typically uses the variable \(p\) for the hypothesis statement, and \(q\) for the conclusion.

 
In words, this would be read as, “If \(p\), then \(q\).”

When the hypothesis and conclusion are identified in a statement, three other statements can be derived:

1. The converse statement is notated as \(q\rightarrow p\) (if \(q\), then \(p\)). The original statements switch positions in the original “if-then” statement.
2. The inverse statement assumes the opposite of each of the original statements and is notated \(\sim p\rightarrow \sim q\) (if not \(p\), then not \(q\)).
3. The contrapositive statement is a combination of the previous two. The positions of \(p\) and \(q\) of the original statement are switched, and then the opposite of each is considered: \(\sim q \rightarrow \sim p\) (if not \(q\), then not \(p\)).

An example will help to make sense of this new terminology and notation. Let’s start with a conditional statement and turn it into our three other statements.

 
The first step is to identify the hypothesis and conclusion statements. Conditional statements make this pretty easy, as the hypothesis follows if and the conclusion follows then. The hypothesis is it is raining and the conclusion is grass is wet.

 
Now we can use the definitions that we introduced earlier to create the three other statements.

• Our converse statement would be: “If the grass is wet, then it is raining.”
• Our inverse statement would be: “If it is NOT raining, then the grass is NOT wet.”
• Our contrapositive statement would be: “If the grass is NOT wet, then it is NOT raining.”

You may be wondering why we would want to go through the trouble of rearranging and considering the “opposite” of the hypothesis and conclusion statements. How is this helpful? The key is in the relationship between the statements. If we know that a statement is true (or false), then we can assume that another is also true (or false). The statements that are related in this way are considered logically equivalent.

For example, consider the statement, “If it is raining, then the grass is wet” to be TRUE. Then you can assume that the contrapositive statement, “If the grass is NOT wet, then it is NOT raining” is also TRUE.

Likewise, the converse statement, “If the grass is wet, then it is raining” is logically equivalent to the inverse statement, “If it is NOT raining, then the grass is NOT wet.”

These relationships are particularly helpful in math courses when you are asked to prove theorems based on definitions that are already known. Much of that work is beyond the scope of this video, but the following examples will help to illustrate the relationships of logically equivalent statements.

Here is a typical example of a TRUE statement that would be made in a geometry class based on the definition of congruent angles:

 
As you can see, this is not a conditional statement, but we can rewrite it in the “if-then” structure to identify the hypothesis and conclusion statements as follows:

 
Now we have a hypothesis and a conclusion.

 
Because the conditional statement and the contrapositive are logically equivalent, we can assume the following to be TRUE:

 
It follows that the converse statement, “If two angles are congruent, then the two angles have the same measure,” is logically equivalent to the inverse statement, “If two angles do NOT have the same measure, then they are NOT congruent.”

Here is another example of a TRUE statement:

 
The conditional statement would be “If a figure is a square, then it is a rectangle,” which gives us our hypothesis and conclusion.

 
Because the contrapositive statement is logically equivalent, we can assume that “If the figure is NOT a rectangle, then the figure is NOT a square” is also a TRUE statement.

However, the converse statement can be disproved.

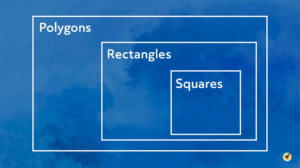
As can be seen in the diagram above, squares are a type of rectangle and a rectangle is a type of polygon. However, a square is a special type of rectangle that has four sides of equal length. Not all rectangles have four equal sides like a square, so our converse statement is FALSE.

Accordingly, the inverse statement is also FALSE because they are logically equivalent:

 
In summary, the original statement is logically equivalent to the contrapositive, and the converse statement is logically equivalent to the inverse.

That is a lot to take in! Let’s end this video with an example for you to process how to analyze a statement to write the converse, inverse, and contrapositive statements.

Review

For this exercise, don’t worry about whether the statements are true or false. The statement is:

 
Now, pause the video and see if you can figure out the converse, inverse, and contrapositive statements. Remember, it helps to first turn our original statement into a conditional statement so you know the hypothesis and conclusion.

Okay, let’s see if you figured it out!

The conditional statement would be: “If all figures are four-sided planes, then figures are rectangles.” This gave us our hypothesis and conclusion.

 
Here are the converse, inverse, and contrapositive statements based on the hypothesis and conclusion:

Converse: “If figures are rectangles, then figures are all four-sided planes.”

Inverse: “If figures are NOT all four-sided planes, then they are NOT rectangles.”

Contrapositive: “If figures are NOT rectangles, then the figures are NOT all four-sided planes.”

That’s all for this review! Thanks for watching, and happy studying!

Converse, Inverse, and Contrapositive Practice Questions