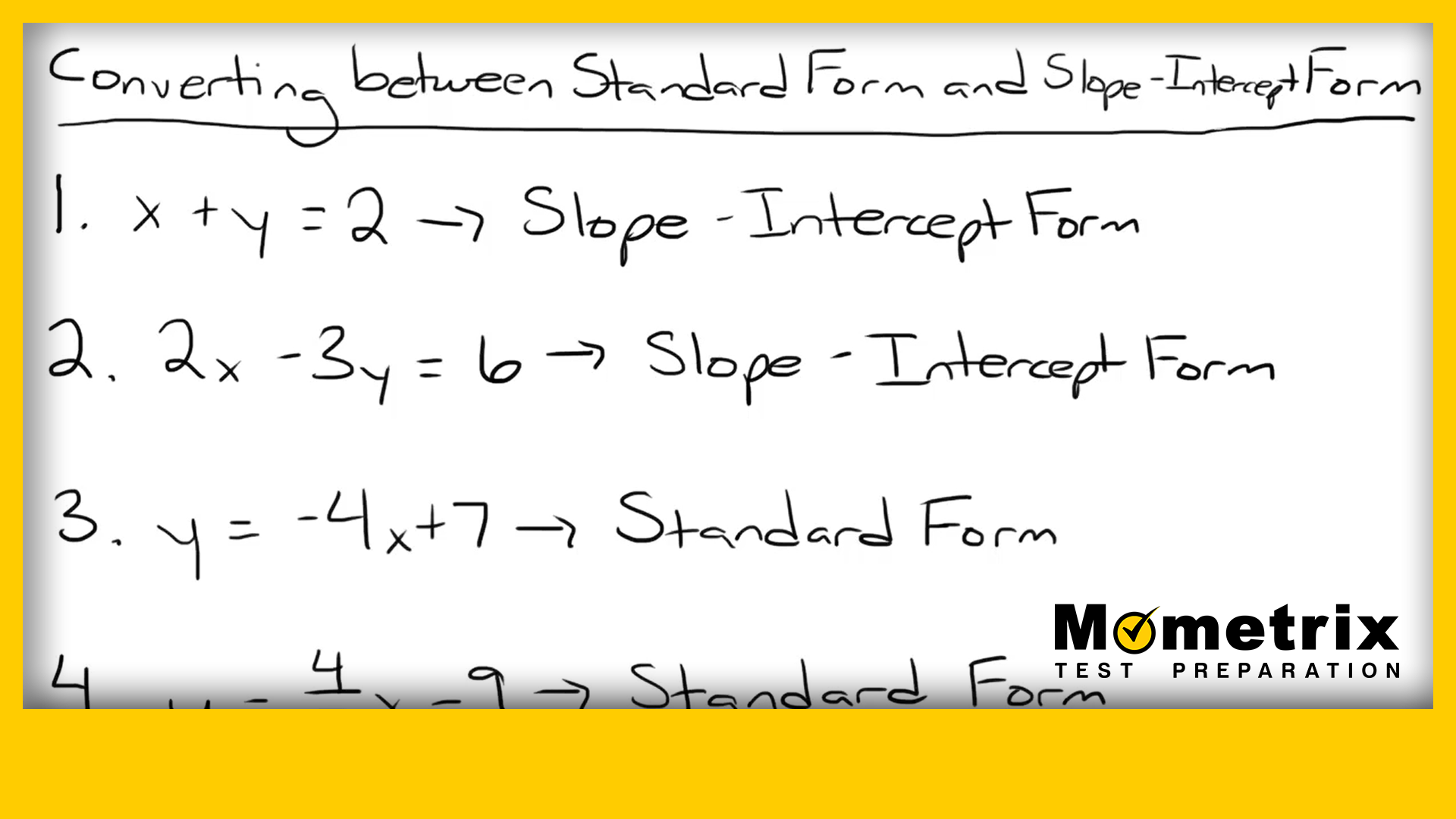
Hello! Today we are going to take a look at how to convert linear equations between standard form and slope-intercept form. As a reminder, the standard form of a linear equation is:
For a standard form equation, \(A\) must be positive, and both \(A\) and \(B\) must be whole numbers. And the slope-intercept form of a linear equation is:
This is called slope-intercept form because the equation itself tells you the slope (\(m\)) and the \(y\)-intercept (\(b\)).
So let’s start with a simple example.
Example #1
Convert the standard form equation \(x+y=2\) to slope-intercept form.
To convert from standard form to slope-intercept form, all we have to do is rearrange our equation to solve for \(y\) and then double check that our terms are in the correct order.
So to do that with this equation, we have to subtract \(x\) from both sides.
When we do that, our \(x\)’s cancel out and we’re left with:
Now, this is almost correct, but remember, slope-intercept form is \(y=mx+b\). That means that our \(x\)-term needs to be first. Because of the commutative property of addition, we know that we can swap our two terms on the right side of our equation. So that will give us:
Notice that I kept the signs with each of the numbers, so we have minus \(x\) up here and that becomes \(-x\), and our 2 is positive up here and that becomes \(+2\). Making sure your signs are correct is super important when you do this. So now, because our equation is in slope-intercept form, we can easily see that our slope, \(m\), is equal to \(-1\), and our \(y\)-intercept, \(b\), is equal to 2.
Let’s try another one!
Example #2
Convert \(2x-3y=6\) to slope-intercept form.
The first thing we need to do is solve our equation for \(y\). We can start by subtracting \(2x\) from both sides of our equation.
So our \(2x\)’s cancel out over here, and we’re left with:
Now, we divide both sides by \(-3\).
We need to divide the entire right side by \(-3\), so this means that each term needs to be divided by \(-3\). It’s easy to want to only divide 6 by \(-3\), but this will give you an incorrect answer. So make sure you also divide \(-2x\) by \(-3\). So over here on our left side, our \(-3\)’s cancel out and we’re left with \(y\) equals, \(6\div (-3)=-2\), because a positive divided by a negative is a negative. And then \(-2x\div (-3)=\frac{2}{3}x\), because a negative divided by a negative is equal to a positive.
So we almost have our correct answer, but we need to switch our terms on the right side. So that will give us:
And we can see that our slope, \(m\), is equal to 23, and our \(y\)-intercept, \(b\), is equal to \(-2\).
But what if we want to go the other way and convert a slope-intercept form equation to standard form? Let’s take a look at an example!
Convert \(y=-4x+7\) to standard form.
Remember, standard form is \(Ax+By=C\). To convert to this form, all we have to do is bring our \(x\)-terms to the left side of the equation, then we’ll make sure our \(A\)-value is positive and both our \(A-\) and \(B-\) values are whole numbers.
So, to move this \(x\)-term to the left side of the equation, for this problem, we’re going to add \(4x\) to both sides.
Our \(4x\)’s will cancel out on the right side, and on the left side we’re left with:
Now we use the commutative property of addition to swap our terms on the left side and get:
Finally, we check to make sure our \(A\)-value is positive and our \(A-\) and \(B\)-values are whole numbers. Both of these things are true, so this is our final answer.
Let’s try one more example.
Example #3
Convert \(y=\frac{4}{7} x-9\) to standard form.
For this equation, we’re going to start by multiplying the entire equation by 7 so that we can get rid of this fractional term..
So, \(7\cdot y=7y\). \(7\cdot \frac{4}{7}x\), our 7’s will cancel out and that’ll leave us with \(4x\). And \(7\cdot 7(-9)=-63\).
So again, we’re going to bring our \(x\)-terms to the left side of the equation by subtracting \(4x\) from both sides.
So that gives us:
Now we’re going to swap our terms on the left side using the commutative property of addition to get:
Finally, we make sure that \(A\) is positive and both \(A\) and \(B\) are whole numbers. \(A\) is negative, so we need to deal with this first. To get rid of the negative in front of \(A\), we multiply the entire equation by \(-1\).
When we do this, we’ll get, \(-1\cdot (-4x)=-4x, -1\cdot 7y=-7y\), and \(-1\cdot (-63)=63\).
Now, our \(A\)-value is positive, and both \(A-\) and \(B\)-values are whole numbers, so this is our final answer.
I hope this video helped you better understand how to convert between standard form and slope-intercept form of linear equations. Thanks for watching and happy studying!
Standard and Slope-Intercept Form Practice Questions
Convert the equation of the line \(y=-4x+\frac{3}{2}\) to standard form.
The equation of a line in standard form is:
\(Ax+By=C\)
- \(A\), \(B\), and \(C\) are integers.
- \(A>0\)
- \(A\) and \(B\) cannot both be zero.
First, let’s clear out the fraction for the equation of our line that is in slope-intercept form by multiplying both sides of the equation by the denominator of the fraction. In this case, multiply both sides of the equation by 2.
\(2\cdot y=2(-4x+\frac{3}{2})\)
\(2y=2(-4x)+2\cdot \frac{3}{2}\)
\(2y=-8x+3\)
Now, move the x-term to the left-hand side of the equation by subtracting \(8x\) from both sides.
\(2y+8x=-8x+3+8x\)
\(8x+2y=3\)
Convert the equation of the line \(2x-7y=14\) to slope-intercept form.
The given equation is in standard form. The equation of a line in slope-intercept form is:
\(y=mx+b\)
- \(m\) is the slope of the line.
- \(b\) is the \(y\)-intercept of the line.
To convert the equation of our line to slope-intercept form, we need to isolate the \(y\)-value. Start by subtracting \(2x\) from both sides.
\(2x-7y-2x=14-2x\)
\(-7y=14-2x\)
Next, divide both sides by –7.
\(\frac{-7y}{-7}=\ \frac{14-2x}{-7}\)
\(y=-2+\frac{2}{7}x\)
Rearranging the terms on the right-hand side of the equation, we get:
\(y=\frac{2}{7}x-2\)
Convert the equation of the line \(y=\frac{3}{5}x-2\) to standard form.
The given equation is in slope-intercept form. The equation of a line in standard form is:
\(Ax+By=C\)
- \(A\), \(B\), and \(C\) are integers.
- \(A>0\)
- \(A\) and \(B\) cannot both be zero.
First, let’s clear out the fraction for the equation of our line by multiplying both sides of the equation by the denominator of the fraction. In this case, multiply both sides of the equation by 5.
\(5\cdot y=5(\frac{3}{5}x-2)\)
\(5y=5\cdot\frac{3}{5}x-5\cdot2\)
\(5y=3x-10\)
Next, we need to be sure the terms containing \(x\) and \(y\) are on the left-hand side of the equation. To do so, subtract \(3x\) from both sides.
\(5y-3x=3x-10-3x\)
\(-3x+5y=-10\)
In the standard form equation of a line, A must be greater than zero. So, multiply both sides of the equation by –1.
\(-1(-3x+5y)=-1\cdot-10\)
\(3x-5y=10\)
You want to join a fitness program. To become a member, there is an initial fee of $75 and a monthly fee of $25. The equation for the total cost can be modeled by the equation \(y=25x+75\), where \(x\) represents the number of months of membership, and \(y\) represents the total cost, in dollars, of being a member. Which of the following is the equation of the total cost of membership in standard form?
The equation of a line in standard form is:
\(Ax+By=C\)
- \(A\), \(B\), and \(C\) are integers.
- \(A>0\)
- \(A\) and \(B\) cannot both be zero.
First, we need to be sure the terms containing \(x\) and \(y\) are on the left-hand side of the equation. So, subtract \(25x\) from both sides of the equation.
\(y-25x=25x+75-25x\)
\(-25x+y=75\)
In the standard form of a line, \(A\) must be greater than zero. So, multiply both sides of the equation by -1.
\(-1(-25x+y)=-1\cdot75\)
\(25x-y=-75\)
You want to produce 10 ounces of an acid solution that is 25% acid. To produce the solution, you mix a 20% acid solution with a 30% acid solution. The equation \(\frac{1}{5}x+\frac{3}{10}y=10\) can be used to represent the number of ounces of the two solutions that are mixed to make the 25% acid solution where \(x\) is the number of ounces of the 20% acid solution and \(y\) is the number of ounces of the 30% acid solution. Which of the following is the equation of the two solutions that are mixed to make the 25% acid solution in slope-intercept form?
The equation of a line in slope-intercept form is:
\(y=mx+b\)
- \(m\) is the slope of the line.
- \(b\) is the \(y\)-intercept of the line.
First, let’s clear out the fraction for the equation of our line by multiplying both sides of the equation by the least common multiple of the two denominators. The least common multiple of 5 and 10 is 10, so multiply both sides of the equation by 10.
\(10(\frac{1}{5}x+\frac{3}{10}y)=10\cdot10\)
\(10\cdot\frac{1}{5}x+10\cdot\frac{3}{10}y=100\)
\(2x+3y=100\)
To convert the equation from standard form to slope-intercept form, we need to isolate the \(y\)-value. Start by subtracting \(2x\) from both sides.
\(2x+3y-2x=100-2x\)
\(3y=100-2x\)
Then, divide both sides of the equation by 3.
\(\frac{3y}{3}=\frac{100-2x}{3}\)
Rearranging the terms on the right-hand side of the equation, we get:
\(y=-\frac{2}{3}x+\frac{100}{3}\)