As you may have already realized through the years of math classes and homework, math is sequential in nature, meaning that each concept is built upon prior work. Arithmetic skills are necessary to conquer algebraic concepts, which are then developed further to be used in calculus, and so on. As you’re building these concepts over time, the math process may become automatic, but the reason, or justification for the work, may be long forgotten.
In this video, we will go back to the basics to review the commutative, associative, and distributive properties of real numbers, which allow for the math mechanics of algebra and beyond.
Commutative Property
The names of the properties that we’re going to be looking at are helpful in decoding their meanings. Consider the word commutative. What do you think of when you see this word? When I look at this word, I see the word commute. That word reminds me of “move,” which is pretty much what the commutative property allows you to do when adding or multiplying algebraic terms. The commutative property looks like this, mathematically:
The commutative property of multiplication: \(a\cdot b=b\cdot a\)
Let’s take a minute to remember the definition of an algebraic term: it is the number, variable, or product of coefficients and variables. Examples of algebraic terms are \(3\), \(3x\), \(3xy\), \(3xy^{2}\), and so on. To prove that moving, or rearranging, terms is acceptable, let’s look at a few examples of the commutative property being used in addition problems.
Example 1
If we add \(5+3\), we get \(8\). But if we switch our terms and make it \(3 + 5\), we still get \(8\). So \(5+3=3+5\).
Example 2
Let’s alter one of our terms a bit for this next example. \(5+(-3)=2\), and \((-3)+5=2\). So \(5+(-3)=(-3)+5\). Note that there is a very important distinction between the addition of a negative integer and the operation of subtraction. It is important to note this distinction because the commutative property does not apply to the operation of subtraction. For instance, \(5-3\) does not yield the same as \(3-5\). This property also does not apply to division. \(100\div 2\neq 2\div 100\).
\(2\div 100=\frac{1}{50}\)
Example 3
The commutative property does, however, apply to multiplication. For example, \(4\times 3\times 5=5\times 3\times 4\). Let’s do the math just to make sure. \(4\times 3=12\times 5=60\). And \(5\times 3=15\times 4=60\). Even though we switched around the terms, we got the same result. Our final example involves the use of variables. Simply substitute values for the variables to show that rearranging terms is acceptable when adding and multiplying. Let’s let \(x=1\), \(y=2\), and \(z=3\). You could substitute with any values, but we’ll use these three for now. So this gives us: \(3(1)^{2}+5(1)(2)+(3)=5(1)(2)+3+3(1)^{2}\)
\(16=16\)
Once we add each side, we are left with 16 on both sides, which is true. \(16=16\).
The next property that we will look at is the associative property.
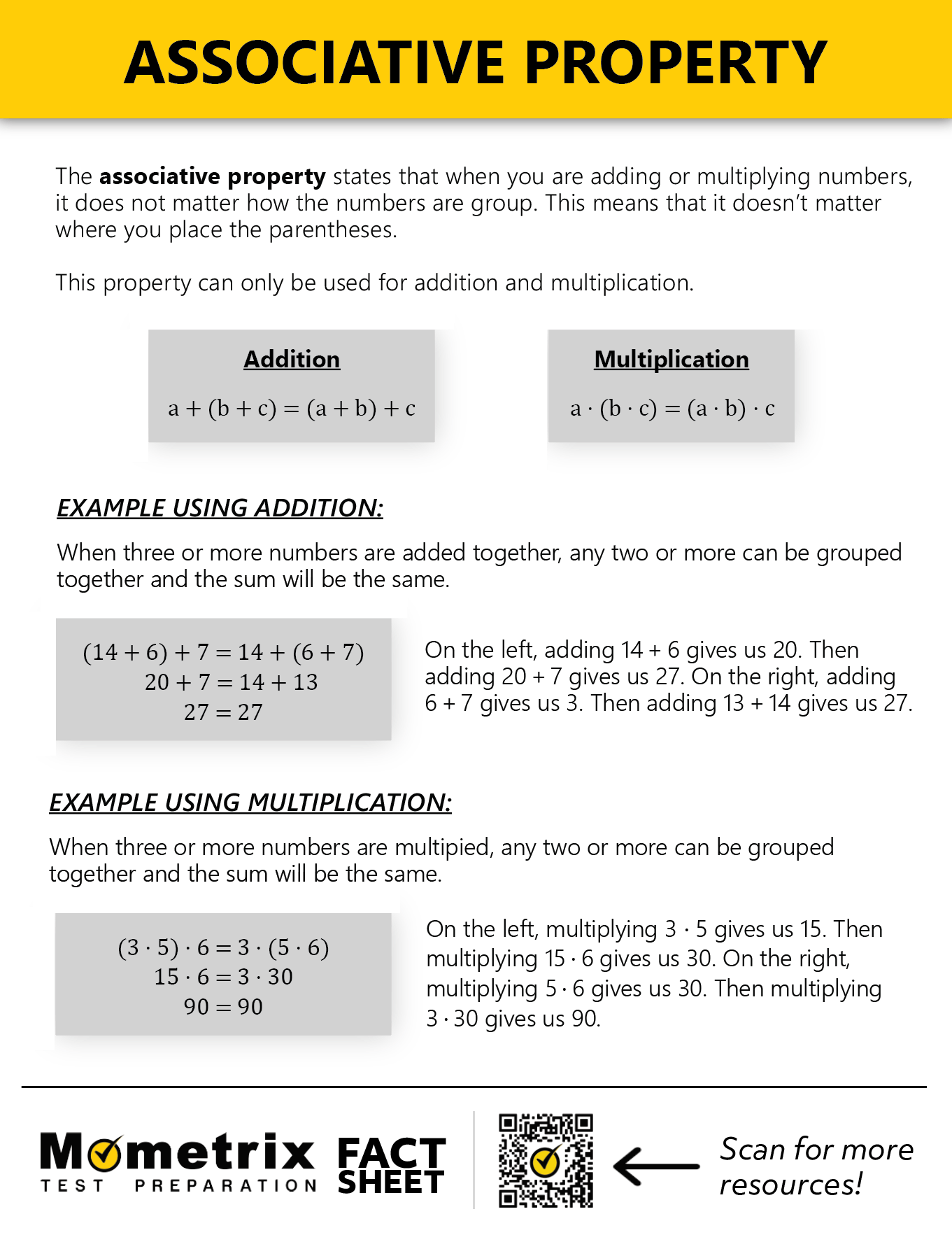
Associative Property
Again, the name provides a helpful hint to its meaning. What comes to mind when you hear the word associative? For me, the word associate stands out, which could also bring to mind the word group. Accordingly, the associative property allows us to group terms that are joined by addition or multiplication in various ways. Parentheses are used to group the terms, and they establish the order of operations. Work inside the parentheses is always done first. Mathematically, the property looks like this:
The associative property of multiplication: \((a\cdot b)\cdot c=a\cdot (b\cdot c)\)
Let’s look at an example of this property used in an addition problem.
Example 1
This example will show that adding the last two terms first or adding the first two terms first simply does not matter. Let’s take a look at \(3+(4+5)=(3+4)+5\). So we do what’s in the parentheses first. \(4+5=9\) and \(9+3=7\).
\(3+9=12\) and \(7+5=12\)
\(12=12\)
So \(12=12\) because it’s on both sides of the equation. Likewise, the order that we perform multiplication does not matter either.
Example 2
Let’s say we have \((3\cdot 4)\cdot 5=3\cdot (4\cdot 5)\).
\(60=60\)
The commutative property of multiplication shows that it is acceptable to rearrange terms when multiplying. In contrast, the associative property of multiplication moves parentheses to order the multiplication.
Distributive Property
Finally, the last property we’ll be looking at is the distributive property, which looks like this: \(a\cdot (b+c)=a\cdot b+a\cdot c\)
The notation, once again, dictates that this property applies only to the operations of multiplication and addition. Specifically, if a term is being multiplied by an expression in parentheses, then the multiplication is performed on each of the terms. Here is an example to prove that this algebraic move is justified. \(2(3+7)=2\cdot 3+2\cdot 7\)
The parentheses on the left tell us to first add 3+7.
\(20=20\)
The sum of the products on the right side of the equation gives the same result as multiplying on the left.
Review
Ok, now that we’ve gone over the three properties, let’s test your memory. For each problem, state the property, commutative, associative, or distributive, that justifies the statement. Go ahead and pause the video if you need more time.
Problem 1:
Problem 2:
Problem 3:
Think you got it? Let’s see! The answer for number 1 is the associative property, because the parentheses are moved to order the multiplication. The answer for number two is the distributive property, because 3 is multiplied by both terms in the parentheses. That leaves us with the answer to number three being the commutative property, because we’ve simply rearranged the terms.
As you can see from our work in this video, you have been using the commutative, associative, and distributive properties for quite some time without even giving the “why” much thought. You will be asked to think about these concepts again in higher-level math courses when some of these properties simply do not hold up! Until then, keep using these rules with confidence to guide your work and thought processes.
I hope this review was helpful. Thanks for watching, and happy studying!
Frequently Asked Questions
Q
What is the commutative property in math?
A
The commutative property applies to addition and multiplication. The property states that terms can “commute,” or move locations, and the result will not be affected. This is expressed as \(a+b=b+a\) for addition, and \(a×b=b×a\) for multiplication. The commutative property does not apply to subtraction or division.
Q
What are 2 examples of the commutative property?
A
The commutative property applies to addition and multiplication. For example, if you have 4 coins in your left pocket and 5 coins in your right pocket, you have 9 coins in all, regardless of which pocket you count first.
\(a+b=b+a\)
\(4+5=5+4\)
The same concept is true for multiplication. For example, in an ice cube tray with 2 rows of 10 cubes, there will be 20 cubes in all, regardless of how you count the cubes. Counting 2 rows of 10, or counting 10 rows of 2 will both generate the same result.
\(a×b=b×a\)
\(2×10=10×2\)
Q
How do you verify the commutative property?
A
The commutative property can be verified using addition or multiplication. This is because the order of terms does not affect the result when adding or multiplying.
For example, when multiplying 5 and 7, the order does not matter. \((5)\times(7)=35\) and \((7)\times(5)=35\). Multiplying 5 chairs per row by 7 rows will give you 35 chairs total, and multiplying 7 chairs per row by 5 rows will also give you 35 chairs total.
Similarly, the order of terms is irrelevant when adding. For example, \((5)+(7)=12\) and \((7)+(5)=12\). If I add 7 blue gumballs to 5 red gumballs, I will have 12 gumballs total. And if I add 5 blue gumballs to 7 red gumballs, I will still have 12 gumballs total.
Q
Is division a commutative property?
A
The commutative property does not apply to division. For example, \(500\div2=250\), but \(2\div500=0.004\). When the terms “commute”, or change locations, the answer changes. In division, the order of the terms matters.
Q
What is an associative property example?
A
The associative property states that when three or more numbers are added or multiplied, and grouping symbols are used, the result will not be affected regardless of where the grouping symbols are located. For example, if you have 5 green marbles, 9 yellow marbles, and 4 blue marbles, you have 18 marbles in all, regardless of which two colors you combine first.
\((a+b)+c=a+(b+c)\)
\((5+9)+4=5+(9+4)\)
\((14)+4=5+(13)\)
\(18=18\)
Similarly, the grouping symbols are somewhat arbitrary when multiplying as well. For example, when calculating the volume of a rectangular prism with a length of 5 in, a width of 4 in, and a height of 3 in, the order that you multiply in does not affect the result. Multiplying the length and the width, and then the height, will produce the same result as multiplying the width and the height, and then the length.
\((a×b)×c=a\times(b×c)\)
\((5×4)×3=5×(4×3)\)
\((20)×3=5×(12)\)
\(60=60\)
Q
What is the associative property formula?
A
The associative property states that when adding or multiplying, the grouping symbols can be relocated without affecting the result. The formula for addition states \((a+b)+c=a+(b+c)\) and the formula for multiplication states \((a×b)×c=a×(b×c)\).
Q
What is the difference between the associative property and the distributive property?
A
The associative property states that when adding or multiplying, the grouping symbols can be rearranged and it will not affect the result. This is stated as \((a+b)+c=a+(b+c)\). The distributive property is a multiplication technique that involves multiplying a number by all the separate addends of another number. This is stated as \(a(b+c)=ab+ac\).
Q
What is the distributive property in math?
A
The distributive property is a method of multiplication where you multiply each addend separately. For example, instead of multiplying \(5\times46\), we can break 46 apart into separate addends \((40+6)\), and multiply 5 by each part separately. \(5\times46\) becomes \(5\times40\) plus \(5\times6\). Essentially the 5 is being “distributed” to each addend.
The distributive property is often used in algebra when simplifying expressions or equations. For example, the distributive property can be used to simplify the expression \(4x(2x^2+6x-9)\). The term \(4x\) will be “distributed” (multiplied) by each term inside the parentheses. \(4x\times2x^2=8x^3\), \(4x\times6x=24x^2\), and \(4x\times-9=-36x\). This means that when \(4x(2x^2+6x-9)\) is “distributed”, the result is \(8x^3+24x^2-36x\). The distributive property can be defined as \(a(b+c)=ab+ac\).
Q
How do you explain the distributive property?
A
The distributive property is formally defined as \(a(b+c)=ab+ac\). The term “distributive property” stems from the term “distribute”. Essentially one number will be “distributed”, or multiplied, by another number that is broken up into separate addends. For example, \(6\times84\) can be solved by “distributing” (multiplying) \(6\times80\) plus \(6\times4\). This property is widely used in algebra when simplifying expressions or equations. For example, \(2x(3x+5)\) can be simplified by distributing (multiplying) the term \(2x\) by each term inside the parentheses \(2x\times3x=6x^2\), and \(2x\times5=10x\). This means that \(2x(3x+5)\) simplifies to \(6x^2+10x\).
Q
What is the commutative property formula?
A
The commutative property formula applies to addition and multiplication. The addition formula states that \(a+b=b+a\), and the multiplication formula states that \(a×b=b×a\). These formulas are used to describe the concept that when adding or multiplying, terms can “commute”, or relocate, and the result will not change.
Q
What is the distributive property in 3rd grade math?
A
The distributive property is a helpful technique for multiplying multi-digit numbers. For example, \(3\times4{,}562\) can seem like a daunting task at first glance. However, if you break apart 4,562 into \(4{,}000+500+60+2\), the pieces become much more manageable. We can now multiply 3 by each of these “pieces”. The distributive property often makes multi-digit multiplication much more manageable.
“Distribute” the 3 to all the addends (multiply).
\(3\times4{,}000=12{,}000\)
\(3\times500=1{,}500\)
\(3\times60=180\)
\(3\times2=6\)
Now add up the pieces. The total is 13,686.
Associative Property of Addition and Multiplication PDF
Commutative, Associative, and Distributive Property Practice Questions
Which of the following is the correct definition of the associative property?
The associative property states that when you are adding or multiplying numbers, it does not matter how the numbers are grouped, meaning it doesn’t matter where you put the parentheses.
Which of the following is a correct example of the associative property?
The correct answer is \((4+3)+(7+11)=4+(3+7)+11\). The associative property says it doesn’t matter how added terms are grouped. Since all these terms are added to one another, the parentheses can be put in any place.
To which of the following operations does the associative property apply?
The correct answer is addition and multiplication. The associative property applies to addition and multiplication but not subtraction and division. Subtraction and division are operations that require being followed in a very specific order, unlike multiplication and division.
Which of the following is not a correct way to rewrite the following expression:
\(4×11×21÷3÷7\)
The correct answer is \(4×11×21÷(3÷7)\). The associative property applies to multiplication but not division, so divided terms cannot be regrouped.
Which of the following is a correct example of the associative property?
The correct answer is \(4×(3×7)=(4×3)×7\). The associative property says you can regroup multiplied terms in any way. Rearranging multiplied terms is an example of the commutative property. Neither of these properties are applicable to division.
Which statement best illustrates the commutative property?
The correct answer is \(6+5=5+6\). The commutative property states that values can be moved or swapped when adding or multiplying, and the outcome will not change. Essentially, the order does not matter when adding or multiplying.
Use the commutative property to find the missing value:
\(45+44+43=43+44+\)_____
The correct answer is 45. The commutative property allows the addition or multiplication of numbers in any order.
Use the commutative property to find the missing values:
\(4+6+\) ____\(=6+\)____ \(+8\)
The correct answer is \(4+6+\mathbf8=6+\mathbf4+8\). Remember, with the commutative property, the order of the numbers does not matter when adding and multiplying.
If \(x=2\), \(y=5\), and \(z=1\), which of the following is true about this equation:
\(2x+4y+9z=9z+4y+2x\)
The correct answer is Both sides are equal to 33.. Even though the terms are listed in a different order, the left and right side of the equation are both equal to 33.
Rewrite the expression \(45+6+19\) using the commutative property.
The correct answer is \(6+45+19\). The expression \(45+6+19\) is equivalent to \(6+45+19\), because changing the order that we add does not affect the result.