Solving Word Problems with Ratios
Hey guys! Welcome to this video tutorial on word problems involving ratios.
Ratios are what we use to compare certain number values. People everywhere use ratios. We use, or at least we should, use ratios when we cook. For example, if I were to make macaroni and cheese for a group of 6 people and I knew that a \(\frac{1}{2}\) cup of macaroni would feed one person, then I could multiply 6 times \(\frac{1}{2}\) to get 3. Well, 3 to 6 is my ratio, and this ratio tells me that for every 3 cups of macaroni that I have, I can serve 6 people.
But hang on. What if I told you that 1 cup of macaroni to every 2 persons is the same ratio as 3:6? Well, it’s the same ratio. 3:6 can be reduced to 1:2 because both 3 and 6 are divisible by 3, which is how we get \(\frac{1}{2}\). So, even though these two ratios look different, they are actually the same.
Let’s take a look at a few word problems, and practice working through them.
Example Problem 1
There are 7 kids in a classroom with green shirts, 8 with red shirts, and 10 with yellow shirts. What is the ratio of people with red and yellow shirts?
- 7:10
- 8:7
- 8:10
- 4:5
Alright, so let’s look at our problem, and see what it is asking us to find, and write out the information that we have been given.
So, there are 7 kids with green shirts; so, let’s write that down. We have Green: 7. We have 8 kids with red shirts, so that is Red: 8, and we have 10 kids with yellow shirts, Yellow: 10.
Now, the question is asking us to find the ratio of kids with red and yellow shirts. This means that we don’t even need to look at our number of green shirts. We’re just looking for the ratio of red shirts to yellow shirts. Well, we have 8 red shirts, and 10 yellow shirts, which gives us a ratio of 8:10.
So now let’s look at each of our options and eliminate. It can’t be 7:10, we don’t care about the green shirts. It can’t be 8:7, because again we don’t care about the green shirts. Option C is correct; that is the exact number ratio we found. Now, look closely at D here. Is 4:5 not the same thing as 8:10? 8 and 10 are both divisible by 2, and when we reduce them both down we get 4:5, so D is also correct!
Great, now let’s look at another word problem.
Example Problem 2
A vegetable tray contains 12 baby carrots, 27 cherry tomatoes, 18 florets of broccoli, and 45 slices of red bell peppers. For every 2 baby carrots, there are 3_____.
Alright, let’s start off the same way that we did our last problem; read through the problem, write down what we know, and find out what is being asked.
So, this vegetable tray contains 12 baby carrots. It contains 27 cherry tomatoes. We have 18 broccoli florets and 45 slices of red bell peppers.
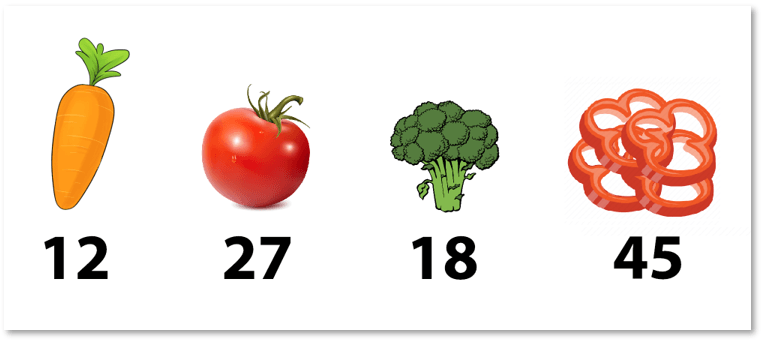
Great, we have all of our information given in the problem, but what are we looking for? It says that for every 2 baby carrots there are 3 somethings. So, we need to find what those somethings are. How do we do that? Well, look at our original number of baby carrots in the problem, it’s 12, but in this ratio, it’s been reduced down to 2. What happened to make this number 2? It was divided by 6. So, since we are dealing with a ratio we know that whatever one number in the ratio was reduced by, the other number has to be reduced in the same way. So, we can multiply 6 times our 3, and 6 times 3 is 18.
When we look at all of our information written down, we can see that we have 18 broccoli florets; so there is our answer. For every 2 baby carrots, there are 3 broccoli florets.
Another way to check and verify that these two ratios are equal is by setting them up in fraction form and cross multiplying.
\(\frac{2}{3}=\frac{12}{18}\)
When we cross multiply, we get \(36=36\).
You can practice finding ratios anywhere you go, like finding the ratio of boys to girls in your class.
I hope that this video helped you to understand how to solve word problems with ratios.
See you guys next time!
Frequently Asked Questions
Q
How do you figure out ratios?
A
A ratio is simply a comparison between two amounts. When figuring out ratios, it is important to consider what two values are being compared. This can be expressed in fraction form, in word form, or simply by using a colon.
When writing a ratio that is comparing a “part” to the “whole”, list the “part” first, and the “whole” second. For example, if you eat \(3\) slices of pizza out of \(10\) slices total, you have eaten \(3\) out of \(10\) slices. This can be expressed as \(\frac{3}{10}\) or \(3\):\(10\), where the part is listed first, and the whole is listed second.
Ratios can also be “part” to “part” comparisons. For example, if there are \(7\) boys in a class, and \(9\) girls in a class, the ratio of boys to girls is \(7\):\(9\). Make sure to match the order of the ratio to the order presented in the scenario.
Q
What are basic ratios?
A
Ratios are used to directly compare two amounts. Basic ratios are used in many real-world situations, which makes it a valuable skill to master. Basic ratios can be expressed as fractions, words, or by using a colon. For example, \(\frac{4}{5}\), “four to five”, and \(4\):\(5\), all represent the same ratio.
Q
What are the 3 ways to write a ratio?
A
A ratio is the comparison between two quantities. There is more than one way to write a ratio. For example, ratios can be written using a fraction bar, using a colon, or using words.
\(\frac{5}{8}\)
\(5\):\(8\)
\(\text{five to eight}\)
Q
What are equivalent ratios?
A
Ratios that have the same value are considered equivalent ratios. For example, if you slice a cake into \(10\) pieces, and you eat \(2\) pieces, you have eaten “\(2\) out of \(10\)” pieces, or \(2\):\(10\). If you had sliced the cake into \(20\) pieces, and eaten \(4\) pieces, you would have eaten the same amount of cake. Eating “4 out of 20” pieces is the same amount as eating “2 out of 10” pieces. Equivalent ratios occur when you multiply or divide both quantities of the ratio by the same amount. \(\frac{50}{100}\) is equivalent to \(\frac{5}{10}\) because when you divide both quantities of the ratio by \(10\), the result is \(\frac{5}{10}\).
Q
How do you find an equivalent ratio?
A
Equivalent ratios can be thought of as equivalent fractions. Two ratios are equivalent if they represent the same amount. For example \(\frac{1}{2}\) and \(\frac{5}{10}\) are equivalent because they represent the same amount. Equivalent ratios are found by multiplying or dividing the numerator and denominator by the same amount. For example, \(3\):\(4\) and \(15\):\(20\) are equivalent ratios because both values in \(3\):\(4\) can be multiplied by \(5\) in order to create the ratio \(15\):\(20\).
Q
How do you solve ratio word problems?
A
Ratios have many real-world applications. Word problems that involve ratios will usually require you to find an unknown value by finding an equivalent ratio.
For example, if you made \($170\) washing \(10\) cars, how much money did you make per car? The ratio of dollars earned compared to cars washed is \(\frac{170}{10}\). We can divide both of these values by \(10\) in order to solve for the dollars earned for washing \(1\) car. \(\frac{170\div10}{10\div10}=\frac{17}{1}\). This means that \($17\) was earned per car. Many word problems involving ratios require you to create equivalent ratios. Remember, as long as you multiply or divide both values of a ratio by the same amount, you have not changed the ratio.
Q
How do you write a ratio in words?
A
Ratios are used to compare two quantities. There are generally two ways to write a ratio in word form. When a ratio is considered a “part-out-of-whole” ratio, the phrase “out of” can be used. For example, if there is a pizza with \(8\) slices, and you eat \(2\) of those slices, you have eaten “\(2\) out of \(8\)” slices. However, some ratios are considered “part-to-part” ratios. For example, when comparing \(3\) green marbles to \(8\) red marbles, the phrase “\(3\) to \(8\)” can be used.
Q
How do you explain ratios and proportions?
A
Ratios describe the relationship between two amounts. Ratios can be described as part-to-part or part-to-whole. For example, in a new litter of puppies, \(4\) of the pups are female and \(3\) of the pups are male. The part-to-part ratio \(4\):\(3\) would be used to compare female to male pups. When comparing female pups to the whole liter, the part-to-whole ratio \(4\):\(7\) would be used. Similarly, the ratio of male pups to total pups would be \(3\):\(7\). If two ratios are equivalent, they are said to be proportional. For example, if \(50\) meters of rope weighs \(5\) kilograms, and \(150\) meters of rope weighs \(15\) kilograms, the two amounts are proportional. \(50\):\(5\) is equivalent to \(150\):\(15\) because both values of the first ratio are multiplied by \(3\) in order to create the second ratio.
Q
What is a ratio, short answer?
A
A ratio is a comparison between two amounts. Ratios can be written using a fraction bar \((\frac{3}{4})\), using a colon (\(3\):\(4\)), or using words (“three to four”). Ratios can be “part-to-part” or “part-to-whole”. For example, \(5\) green marbles and \(7\) red marbles would be a “part-to-part” ratio (\(5\):\(7\)). Solving \(95\) problems correctly out of \(100\) on a math test would be a “part-to-whole” ratio (\(95\):\(100\)).
Ratio Word Problems
Cross multiply in order to determine which pair of ratios are equivalent.
3:5 and 4:9
6:7 and 7:8
7:8 and 35:40
12:25 and 14:17
The correct answer is 7:8 and 35:40.
We can use cross multiplication to determine if two ratios are equivalent. Let’s look at the ratios for Choice C, and let’s set these up as fractions:
\(\frac{7}{8}\) and \(\frac{35}{40}\)
Now, cross multiply by finding the product of \(8×35\) and \(7×40\). In both cases our product is 280, so we know that the original ratios are equivalent.
Which ratio is equivalent to \(\frac{36}{45}\)?
5:6
4:5
The correct answer is 4:5.
We can simplify ratios with the same strategy that we use to simplify fractions. In the ratio \(\frac{36}{45}\) we can divide the numerator and denominator by 9. \(\frac{36}{45}\) now becomes \(\frac{4}{5}\) or 4:5.
James has a bag full of red, blue, and yellow candy. There are 10 red candies, 9 blue candies, and 11 yellow candies. What is the ratio of blue candies to total candies in the bag? Simplify the ratio if possible.
3:10
9:35
4:11
10:30
The correct answer is 3:10.
The total number of blue candies is 9, and the total number of candies in the bag is 30. If we set this ratio up as a fraction, we have \(\frac{9}{30}\). This fraction can be simplified if we divide the numerator and denominator by 3. Our final answer is \(\frac{3}{10}\) or 3:10.
Alex is counting the coins in his pocket, and finds that he has 14 quarters, 7 nickels, and 4 dimes. What is the ratio of quarters to nickels? Simplify if possible.
4:7
14:7
1:3
2:1
The correct answer is 2:1.
We can compare the number of quarters to the number of nickels by setting up a ratio. There are 14 quarters and 7 nickels, so our ratio would be 14:7. Choice B says 14:7, but we should simplify when possible. 14:7 simplifies to 2:1.
In Mr. Jenkin’s 4th grade class there are 14 boys and 17 girls. What is the ratio of boys to girls? Simplify if possible.
17:12
14:7
14:17
7:11
The correct answer is 14:17.
We can express this comparison of boys to girls as a ratio. 14 boys and 17 girls can be described as the ratio 14:17, or the fraction \(\frac{14}{17}\). 14 and 17 do not have any factors in common, so 14:17 is in simplest form.