
Hi, and welcome to this video on circles! The circle is one of the first shapes we learn and one we see every day all around us. Its elegant simplicity is even reflected in its formulas for finding the circumference and area of any circle:
Circumference and Area Formulas
\(A=πr^2\)
Using these simple formulas does require us to define a few terms so we can solve circle problems.
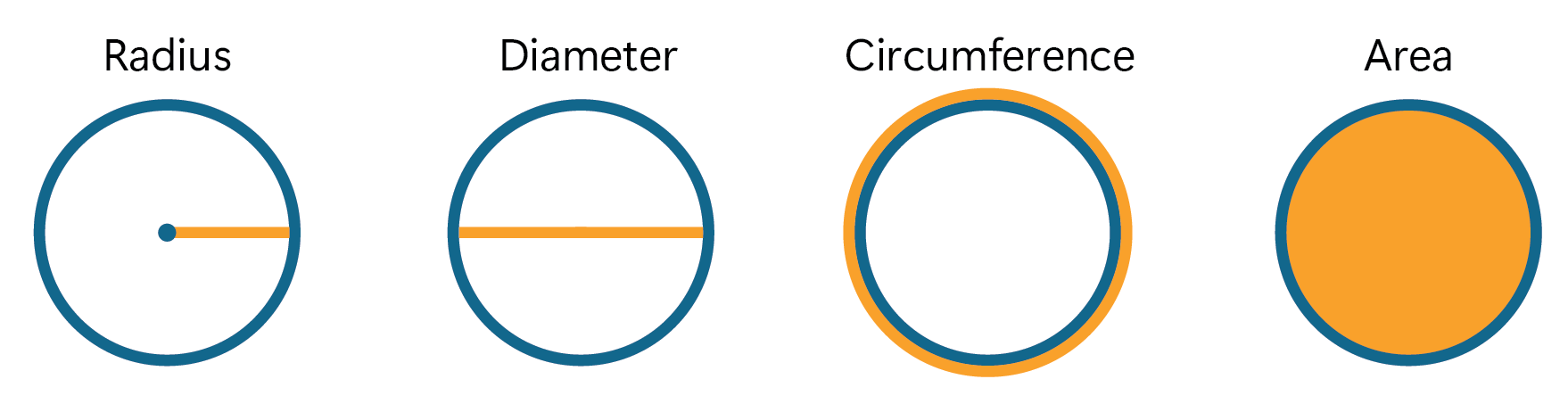
Radius
First, we need to define the radius, which is the \(r\) in our two formulas. The radius is the distance from the center of the circle to any point on the outside of the circle. If we were drawing a circle using a string tied to a pencil, the radius would be the length of the string.
Diameter
Next up is the diameter, which doesn’t appear in either of our formulas. But it’s a very important part of a circle, especially for real-world problems. The diameter is the distance all the way across a circle when going through the center. It’s always exactly twice as long as the radius. In fact, there are versions of the circumference and area formulas that use the diameter instead of the radius:
\(A=\frac{πd^{2}}{4}\)
In practice, this area formula isn’t used as often as the radius formula, but it does work. When we solve a problem where the diameter is known, we usually just divide the diameter by 2 to find the radius and then use the radius formulas.
Circumference
The big \(C\) in the formulas is the circumference. This is the distance around a circle. It’s like the perimeter of polygons and it’s measured in length units like meters and feet. If we were building a circular fence around a yard, you’d need to know the circumference of the circle to know how many feet of fence to buy.
Area
The big \(A\) in the formulas is the area of the circle. This is the measure of how many square units, such as square centimeters or square inches, will fit inside the circle. If we wanted to paint a large solid circle as part of a mural, we’d need to know the area of the circle to calculate how much paint we would need to buy to fill in the whole circle.
Pi
Finally, we need to define pi. Pi is a number. We sometimes use 3.14 as an approximation of pi, since that will get us close to the real answer. The reality is that pi is an irrational number, which means it can’t be written as a fraction. That also means that it’s a decimal that goes on forever but doesn’t repeat.
So whenever we use 3.14, or even that pi key on a calculator, we’re using an approximation of pi and our answer will also be an approximation. Exact answers will keep pi as part of the answer, but for real-world problems we use the approximate answer since it’s more useful when buying things like fencing or paint. No one sells those things in quantities of pi!
Solving Real-World Problems
Let’s use our knowledge of these terms to solve some real-world problems.
Problem #1
The town hall has a giant clock that needs some maintenance. The clock face needs to be removed and painted, and it needs new rubber edging all around the outside to stop water from going inside. The clock measures 4.5 meters high. How many square meters do we need to paint, and how many meters of edging do we need to buy?
Let’s look at our two formulas again.
\(A=πr^2\)
The painting part of the problem is our area. The edging is the circumference. We know the height of our clock face, which is the diameter. Let’s calculate them one at a time, starting with the area.
The first thing we need to do is find the radius from the diameter, which we do by dividing by 2.
\(r=\frac{4.5\text{ m}}{2}\)
\(r=2.25 m\)
Now that we know the radius, we can plug it into our area formula.
\(A=π(2.25 m)^2\)
\(A=5.0625\pi\text{ m}^2\)
This is the exact answer in terms of pi but not very useful for real-world applications. Multiplying by our calculator approximation of pi gives us:
So just under 16 square meters. We need to find a can of paint that can cover that many square meters.
Now what about our rubber edging? Let’s find the circumference:
\(C=2π(2.25\text{ m})\)
\(C=4.5π\text{ m}\)
Once again, we have our exact answer in terms of pi. Using our calculator, we get our approximate answer:
We’ll need to buy a roll of rubber edging that is more than 14.14 meters to maintain our clock.
Notice that circumference is a measure of distance. Here it’s measured in meters. This can be very useful for finding distances when using wheeled vehicles.
Problem #2
For instance, if we want to measure how far we travel on a bicycle, we can use the circumference of the tires to help us.
How far will we travel on our BMX bicycle when we ride around the block? We do the old playing card in the spokes trick so that the card will make a sound each time it hits the fork of the bike. Then we count the number of times the sound happened as we rode around the block. We count 212 “clicks.” What else do we need to know? The circumference of the tire!
It’s not easy to measure the circumference of a tire, but we don’t need to. We can measure the height of the tire, which is also the diameter, then use a formula to calculate the circumference.
Our bike uses 20” wheels, but when we actually measure the height of the tire, it’s 24 inches, or 2 feet. This is the diameter. Let’s use the diameter version of the circumference formula this time instead of the radius version:
\(C=π(2\text{ft})\)
\(C=2π\text{ ft}\)
This is how far the bike travels each time the tire does one rotation. To find our distance, we simply need to multiply this by the number of times the tire fully rotated, which is 212:
\(\text{ Distance}=424π\text{ ft}\)
\(\text{ Distance}\approx{1,332}\text{ ft}\)
Review
Before we go, I want you to try one on your own. We have a square yard that is 30 × 30 meters. We want to plant grass in a circle in the center of the yard that measures 20 meters across. How many square meters of grass will we need to cover with sod?
Pause the video here and try it on your own. When you’re ready, press play and we’ll go over it together.
First we need to find the radius. The circle is 20 meters across, which is the diameter. The radius is half of that, so 10 meters. Now we just plug that into our formula:
\(A=π(10 m)^2\)
\(A=100π m^2\)
Finally, we multiply by on our calculator to get our approximate area:
We need to buy a lot of sod!
That’s it for the area and circumference of circles. Thanks for watching, and happy studying!
Frequently Asked Questions
Q
How do you find the area of a circle?
A
Find the area of a circle by squaring its radius and multiplying by pi (π).
A = πr2
Ex. What is the area of a circle with radius 4 cm?
A = πr2 = π(4)2 = 16π cm2 ≈ 50.27 cm2
Q
How do you find the circumference of a circle?
A
Find the circumference of a circle by multiplying its diameter by pi (π) or doubling its radius and multiplying by pi.
C = πd or C = 2πr
Ex. What is the circumference of a circle with a radius of 2 cm?
C = 2πr = 2π(2) = 4π cm ≈ 12.56 cm
Q
How do you find the diameter of a circle?
A
Find the diameter of a circle by measuring the distance between two of the furthest points on a circle. The diameter always passes through the center of the circle. 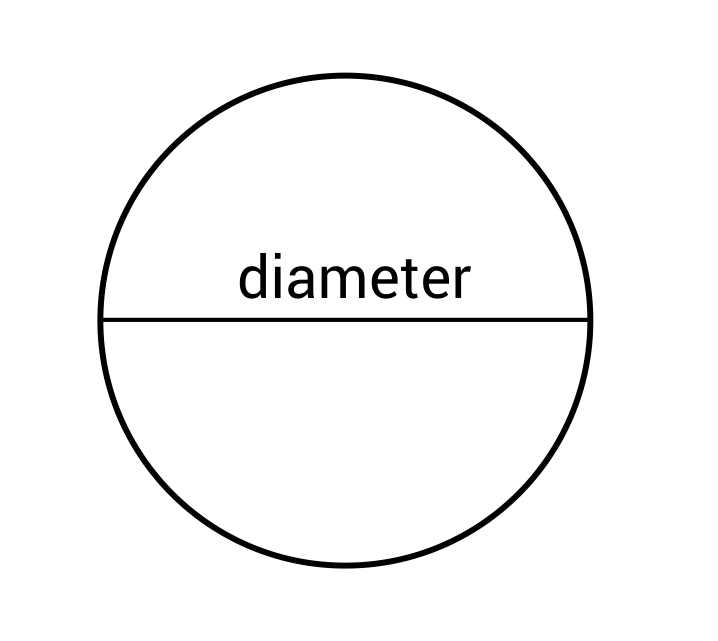
Q
How do you find the radius of a circle?
A
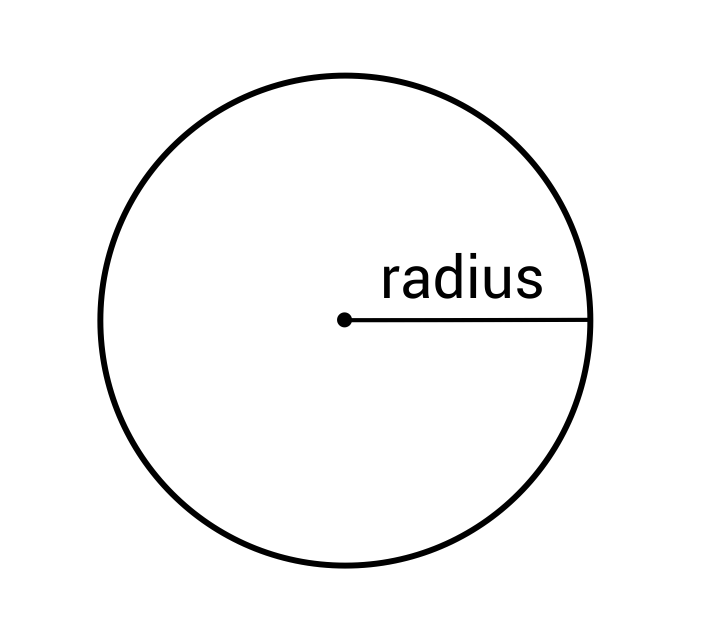
Find the radius of a circle by connecting the center of the circle to any point on the circle, or by taking half the diameter.
Q
What is pi?
A
Pi (π) is a Greek letter that represents the ratio of a circle’s circumference to its diameter. It is a never-ending number that cannot be written as a fraction, which means it is irrational. The approximate value of pi (π) is 3.14.
Area and Circumference of a Circle Practice Questions
What is the area of a circle that has a diameter of 12 centimeters? Use 3.14 for π.
The area of a circle is \(A=πr^2\), where \(r\) is the radius of the circle. If it is not given, we can find the value of the radius if the diameter of the circle is given.
\(r=\frac{d}{2}\)
Since the diameter of our circle is 12 cm, we have:
\(r=\frac{12}{2}=6\)
Substituting the value of the radius into the formula for the area of a circle, we get:
\(A=3.14(6)^2=3.14(36)=113.04\)
So, the area of the circle is \(113.04\text{ cm}^2\).
What is the area of a circle that has a circumference of 50.24 inches? Use 3.14 for π.
The area of a circle is \(A=πr^2\), where r is the radius of the circle. We are not given the radius of the circle but we can use the given circumference of the circle to find it. The circumference of a circle is:
\(C=2r\)
Since the circumference of our circle is 50.24 inches, we have:
\(50.24=2(3.14)r\)
Solving for r, we get:
\(50.24=23.14r\)
\(50.24=6.28r\)
\(\frac{50.24}{6.28}=\frac{6.28r}{6.28}\)
\(r=8\)
Substituting the value of the radius into the formula for the area of a circle, we have:
\(A=3.14(8)^2=3.14(64)=200.96\)
So, the area of the circle is \(200.96\text{ in}^2\).
The area of a circle is \(452.16\text{ ft}^2\). What is the circumference of the circle? Use 3.14 for π.
The circumference of a circle is \(C=2πr\), where \(r\) is the radius of the circle.
We are not given the radius of the circle but we can use the given area of the circle to find it. The area of a circle is:
\(A=πr^2\)
Since the area of our circle is 452.16 square inches, we have:
\(452.16=3.14r^2\)
Solving for \(r\), we get:
\(\frac{452.16}{3.14}=\frac{3.14∙r^2}{3.14}\)
\(144=r^2\)
\(\sqrt{144}=\sqrt{r^2}\)
\(r=12\)
*Taking the square root 144 results in \(\pm12\), but since length values can’t be negative, we only consider positive 12.*
Substituting the value of the radius into the formula for the circumference of a circle, we have:
\(C=2(3.14)12=75.36\)
So, the circumference of the circle is 75.36 ft.
Suppose the circumference for the center circle of a soccer field is 56.52 yards. If the center circle is to be painted with a fast-drying acrylic paint, how much paint, in square feet, will be needed? Use 3.14 for π.
We need to find the space contained inside the center circle to determine how much paint is needed, so we need to find the area of the center circle. The area of a circle is \(A=πr^2\), where \(r\) is the radius of the circle. We are not given the radius of the center circle but we can use the given circumference of the circle to find it.
The circumference of a circle is:
\(C=2πr\)
Since the circumference of our center circle is 56.52 feet, we have:
\(56.52=2(3.14)r\)
Solving for \(r\), we get:
\(56.52=2(3.14)r\)
\(56.52=6.28r\)
\(\frac{56.52}{6.28}=\frac{6.28r}{6.28}\)
\(r=9\)
Substituting the value of the radius into the formula for the area of a circle, we have:
\(A=3.14(9)2=3.14(81)=254.34\)
So, \(254.34\text{ ft}^2\) of paint will be needed to paint the center circle of the soccer field.
A circular fence is to be built around a garden to protect it from animals digging it up. The area of the garden that needs to be fenced in is \(706.5\text{ ft}^2\). How much fencing is needed to protect the garden? Use 3.14 for π.
Since we need to find the amount of fencing needed to protect the circular garden, we need to find the circumference of the garden. The circumference of a circle is \(C=2πr\), where \(r\) is the radius of the circle.
We are not given the radius of the circular garden but we can use the given area of the circle to find it. The area of a circle is:
\(A=πr^2\)
Since the area of our circle is 706.5 square feet, we have:
\(706.5=3.14r^2\)
Solving for \(r\), we get:
\(\frac{706.5}{3.14}=\frac{3.14∙r^2}{3.14}\)
\(225=r^2\)
\(\sqrt{225}=\sqrt{r^2}\)
\(r=15\)
*Taking the square root 225 results in \(\pm15\), but since length values can’t be negative, we only consider positive 15.*
Substituting the value of the radius into the formula for the circumference of a circle, we have:
\(C=2(3.14)(15)=94.2\)
So, the circumference of the circle is 94.2 ft.